maximum volume of box with 20m 2 of sheet metal 5, or 20 inches. Realizar la estructura sin soldadura hace que sea un proceso seguro, limpio y rápido. Se complementa con elementos adicionales para solventar soluciones constructivas de fachada, cubiertas, puertas o remates.
0 · maximum volume of a box
1 · how to calculate maximum volume
2 · how to calculate maximum box volume
3 · cut out box volume
4 · calculus box volume chart
5 · calculate volume of boxes
6 · calculate box volume when corners
7 · box volume when corners cut out
Page 1 of 3 JB1706 Underwater Junction Box HIGHLIGHTS • Designed for connection of supply cords from underwater fixtures and service conduits • Heavy wall cast bronze • Stainless steel .
Optimization: Maximizing volume. One of the key applications of finding global extrema is in optimizing some quantity, either minimizing or maximizing it. For example, suppose you wanted to make an open-topped box out of a flat piece of cardboard that is 25" long by 20" wide. 5, or 20 inches.
Suppose that the box height is $h = 3 in.$ and that it is constructed using 4 in.^2$ of cardboard (i.e., $AB = 134$). Which values $A$ and $B$ maximize the volume? How do I approach this question when there are the . To optimize the volume of a box, you can adjust the dimensions of the box to increase or decrease the volume. Keeping the dimensions proportional to each other will result .To do so, he cuts 4 equal sized squares from the corners of the sheet and then folds the remaining metal upwards to create the sides of a box. What is the maximum possible volume . You are planning to make an open box from a 30- by 42 inch piece of sheet metal by cutting congruent squares from the corners and folding up the sides. You want the box to .
A rectangular sheet of metal having dimensions 20 cm by 12 cm has squares removed from each of the four corners and the sides bent upwards to form an open box. Determine the maximum possible volume of the box.
3) A closed rectangular container with square ends (as shown below) needs to be constructed using a metal sheet. a) Define the surface area of the container in terms of x and y. b) Define the volume of the container in terms of x and y. c) .
An open rectangular box with square ends is fitted with an overlapping lid which covers the top and the front face. Determine the maximum volume of the box if 6 m² of metal are used in its .VIDEO ANSWER: An open rectangular box is to be manufactured from a given amount of sheet metal (area S ). Find the dimensions of the box to maximize the volume.An open box is to be constructed by cutting equal squares from a rectangular piece of metal. Find the dimensions of the box of maximum volume if the piece of metal is 5 by 8. Determine what the maximu; A 6'6" square sheet of metal is made into an open box by cutting out a square at each corner and then folding up the four sides.
The maximum amount of bend angles between pull points in a conduit run cannot exceed_____. 360 degrees . ____ Sheet-metal boxes are the most commonly used boxes in industrial and commercial applicants . Fill. Box _____ is the volume occupied by conductors, components, and devices. Driptight. A ____ enclosure is an enclosure that does not .Question: 2. A rectangular sheet of metal having dimensions 20cm by 12cm has squares removed from each of the four corners and the sides bent upwards to form an open box. Determine the maximum possible volume of the box. 3. The distance x meters travelled by a vehicle in time t seconds after the brakes are applied is given by: x = 20t - t?.
If the volume of the box is maximum, the dimensions of the box are. Q. An open tank with a square base and vertical side is to be constructed a metal sheet so as to hold a given quantity of water. Show that the cost of the material will be least when depth of the tank is half of its width.
A box without lid having maximum possible volume is to be made from a rectangular piece of tin 32 cm x 20 cms by cutting of equal square pieces from the four corners and turning up the projecting pieces to make the sides of the box.The side of the base such that the volume of this tank is maximum, is. Login. Study Materials. NCERT Solutions. NCERT Solutions For Class 12. NCERT Solutions For Class 12 Physics; . If 40 square feet of sheet metal are to be used in the construction of an open tank with square base, find the maximum capacity of the tank.
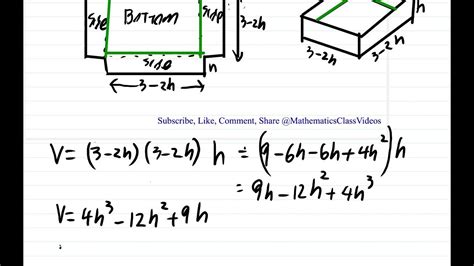
A rectangular sheet of metal 750 mm \times 300 mm - find the length of the sides of the squares to give maximum volume to the box, and find the maximum volume of the box? From a thin piece of cardboard 50 in. by 50 in., square corners are cut out so that the sides can be folded up to make a box. What dimensions will yield a box of maximum volume? Maximum volume of the box will be 7.41 cubic feet. Step-by-step explanation: Open box has been made from a metal sheet measuring 3 ft and 8 ft. Let four square pieces were removed from the four corners with one side measurement x ft. Volume of the open box = Length × width × height. Length of the box = (3 - 2x) ft. Width of the box = (8 - 2x) ft
Determine the maximum possible volume of the box. A rectangular sheet of metal having dimensions 20 cm by 12 cm has squares removed from each of the four corners and the sides bent upwards to form an open box. Determine the maximum possible volume of the box. The volume of a box is given by length x width x height. In this case, it will be: V = (20-2x)(12-2x)x. To find the maximum volume, we should differentiate this volume equation with respect to x and set the derivative equal to zero. Solving it, we find x=2 cm. Substituting x=2 into the volume equation, we get V = (20-4)(12-4)2 = 14.4 * 4 = 57.6 .
maximum volume of a box
A 6" x 6" square sheet of metal is made into an open box by cutting out a square at each corner and then folding up the four sides. Determine the maximum volume, Vmax, of the box. 1. Vmax = 16 cu. ins. 2. Vmax = 31 cu. ins. 3. Vmax = 21 cu. ins. 4. Vmax = 26 cu. ins. 5. Vmax 36 cu, ins.A rectangular sheet of the fixed perimeter with sides having their lengths in the ratio 8: 15 is converted into an open rectangular box by folding after removing squares of the equal area from all four corners. If the total area of removed squares is 100, the resulting box has maximum volume. The lengths of the sides of the rectangular sheet areAnswer to 2) A metal box (without a top) is to be constructed. Math; Calculus; Calculus questions and answers; 2) A metal box (without a top) is to be constructed from a square sheet of metal that is 20 cm on a side by cutting .16. You are given a piece of sheet metal that is twice as long as it is wide and has an area of 800 m². Find the dimensions of the rectangular box that would contain a maximum volume if it were constructed from this piece of metal by cutting out squares of equal area at all four corners and folding up the sides. The box will not have a lid.
A rectangular sheet of metal with dimensions 20 cm by 15 cm is to be used to create an open top box by cutting a square, a cm by z cm, from each comer and bending up the sides. Ita volume of 375 cm is required, determine the side . Squares are cut from each corner. The sides are then folded to make a box. Find the maximum volume of the box. 2. An open box is made from a thin sheet of cardboard with sides 15 cm by 10 cm. Squares are cut from each corner. The sides are then folded to make a box. Find the maximum volume of the box.A square piece of tin of side 18 cm is to be made into a box without top by cutting a square from each corner and folding up the flaps to form a box. What should be the side of the square to be cut off so that the volume of the box is maximum? Find this maximum volume. The maximum volume of the box that can be constructed from the square sheet of metal is V = (x/2)^2 * (x - 2(x/2)) = x^3/8. How to calculate the maximum volume of the box? Let's assume that the original square sheet of metal has a side length of 'x'. We will cut squares of length 'y' from each corner of the sheet to form the metal box.
A box with a square base and an open top is to be made. You have 00\operatorname{cm}^2$ of material to make it. What is the maximum volume the box could have? Here's what I did: $00 = x^2+. The volume of the box can be written in the form: "V(x)=(L - 2\cdot x)\cdot(W- 2\cdot x)\cdot x" Lengths and width of the box decreased that is of sheet metal by "x" from each corner, and height of the box is equal "x".We bring "V(x)" to a simple form: "V(x)=4\cdot x^3 -2\cdot (L+W)\cdot x^2+ L\cdot W\cdot x". To find maximum volume one compute the .Determine the maximum possible volume of the box. A rectangular sheet of metal having dimensions \mathrm{~cm}$ by \mathrm{~cm}$ has squares removed from each of the four corners and the sides bent upwards to form an open box. Determine the maximum possible volume of the box. Show more.

4x4 metal combo electric box
A rectangular sheet of metal having dimensions 20 cm by 12 cm has squares removed from each of the four corners and the sides bent upwards to form an open box. Determine the maximum possible volume of the box.A box is being constructed by cutting 2 2 2-inch squares from the corners of a square sheet of metal. If the box is to have a volume of 1058 1058 1058 cubic inches, find the dimensions of the metal sheet.The extremum (dig that fancy word for maximum or minimum) you’re looking for doesn’t often occur at an endpoint, but it can — so don’t fail to evaluate the function at the interval’s two endpoints.. You’ve got your answer: a height of 5 inches produces the box with maximum volume (2000 cubic inches). Because the length and width equal 30 – 2h, a height of 5 inches gives a .
2) A metal box (without a top) is to be constructed from a square sheet of metal that is 20 cm on a side by cutting square pieces of the same size from the corners of the sheet and then folding up the sides. Find the dimensions of the box with the largest volume that can be constructed in this manner. ! V(x)=x(20"2x)(20"2x)=400x"80x2+4x3
4x2 steel box section
Quazite products are tested to meet ANSI/SCTE specifications for underground enclosure integrity and are manufactured in Lenoir City, Tennessee. Polymer concrete is made from selectively-graded aggregates in combination with a polymer resin system.Wide Usability :Suitable for fast connection to the outdoor lighting, underfloor heating, beacon, watering cart, solar photovaltaic, cruise, etc. Can be used to repair and extend power cables.
maximum volume of box with 20m 2 of sheet metal|how to calculate maximum box volume