distribution balls into boxes java There are x boxes and y balls where x <= y, and I want to distribute the balls to put them inside the boxes in order. For example: 3 boxes; box A, box B and box C - and 5 balls; . Wiring diagrams led me to believe that there were differences between N54 and N55 JBE's but if you check RealOEM, its the same module from like 2006 to 2012. I've attached a pic of the little rat bastard. It's located behind the glove box and just below the fuse block.There is NO box 12a on the 1099-R but Box 14 labelled state tax withheld, which TT has highlighted, is greater than empty Box 15 titled State distribution. Additionally, the amount highlighted in Box 14 IS less than box 1 Gross distribution.
0 · java
1 · Enumerating the ways of distributing n balls into k boxes
2 · Distributing balls into bins randomly
3 · Distributing balls in boxes.
4 · Distributing all balls without repetition
5 · Distributing Balls
6 · Balls in Boxes
7 · Algorithms for allocating N balls into M boxes
8 · 3074. Apple Redistribution into Boxes
Owner/electrical contractor Feb 15, 2020 . Still wouldn’t make it compliant. (Picture of a 1900 box)
Choose a way to represent the distribution of balls into boxes (e.g. an array, with the ball as the index and the box as the value). Write a function isDistributionValid(distribution) .
There are x boxes and y balls where x <= y, and I want to distribute the balls to put them inside the boxes in order. For example: 3 boxes; box A, box B and box C - and 5 balls; .
java
Enumerating the ways of distributing n balls into k boxes
Given an array arr[] of size N, representing the number of balls of each of N distinct colors, the task is to find the probability of distributing all the balls into two boxes, such that . Every box has at least no balls and at most N balls. Of course the total number of balls in M boxes must equal to N. For each allocation, I calculated a value based on the . Enumerate the ways of distributing the balls into boxes. Some boxes may be empty. We can represent each distribution in the form of n stars and k − 1 vertical lines. The .Each box has a set of 10 distinct colored balls. The colors are numbered from 1 to 10. Each box has a cost associated with it. She is required to spend the minimum amount of money to buy .
Geek wants to distribute M balls among N children. His nemesis Keeg wants to disrupt his plan and removes P balls from Geek's bag. The minimum number of balls required to make each .Now, you can distribute apples from any single pack into multiple boxes if necessary, but what you're trying to find out is the smallest number of boxes you can use to hold all the apples. . If $n$ balls are distributed at random into $r$ boxes (where $r \geq 3$), what is the probability that box $ at exactly $j$ balls for In how many ways can $n$ identical balls be distributed amongst $m$ different boxes given that a box can have any number of balls(from Choose a way to represent the distribution of balls into boxes (e.g. an array, with the ball as the index and the box as the value). Write a function isDistributionValid(distribution) that takes a distribution and returns true if all the constraints are satisfied.$ to $n$)? What I've tried is using multinomial . \leq j \leq n$ and box $ contains exactly . There are x boxes and y balls where x <= y, and I want to distribute the balls to put them inside the boxes in order. For example: 3 boxes; box A, box B and box C - and 5 balls; ball 1, ball 2, ball 3, ball 4, ball 5.
You can define your function assuming the limits c[0], c[1], . c[m-1] as fixed and then writing the recursive formula that returns the number of ways you can distribute n balls into bins starting at index k. With this approach a basic formula is simply. if n == 0: return 1 # Out of balls, there's only one solution (0, 0, 0, 0 . 0) if k == m: Given an array arr[] of size N, representing the number of balls of each of N distinct colors, the task is to find the probability of distributing all the balls into two boxes, such that both the boxes contain an equal number of distinct colored balls. Every box has at least no balls and at most N balls. Of course the total number of balls in M boxes must equal to N. For each allocation, I calculated a value based on the allocation: V=f(n_1,n_2,.n_m) .
Enumerate the ways of distributing the balls into boxes. Some boxes may be empty. We can represent each distribution in the form of n stars and k − 1 vertical lines. The stars represent balls, and the vertical lines divide the balls into boxes. For example, here are the possible distributions for n = 3, k = 3:Each box has a set of 10 distinct colored balls. The colors are numbered from 1 to 10. Each box has a cost associated with it. She is required to spend the minimum amount of money to buy the boxes such that she has maximum distinct colored balls in the end. Input format.Geek wants to distribute M balls among N children. His nemesis Keeg wants to disrupt his plan and removes P balls from Geek's bag. The minimum number of balls required to make each child happy are given in an array arr[]. Find the number of ways
Now, you can distribute apples from any single pack into multiple boxes if necessary, but what you're trying to find out is the smallest number of boxes you can use to hold all the apples. Imagine you're moving and have a collection of differently sized boxes and many items of .This snippet implements your example of distributing 5 balls into baskets of size 2, 3, and 2. The starting polynomial basket(0) is just the constant 1 and is a neutral element for polynomial multiplication. Choose a way to represent the distribution of balls into boxes (e.g. an array, with the ball as the index and the box as the value). Write a function isDistributionValid(distribution) that takes a distribution and returns true if all the constraints are satisfied. There are x boxes and y balls where x <= y, and I want to distribute the balls to put them inside the boxes in order. For example: 3 boxes; box A, box B and box C - and 5 balls; ball 1, ball 2, ball 3, ball 4, ball 5.
Distributing balls into bins randomly
You can define your function assuming the limits c[0], c[1], . c[m-1] as fixed and then writing the recursive formula that returns the number of ways you can distribute n balls into bins starting at index k. With this approach a basic formula is simply. if n == 0: return 1 # Out of balls, there's only one solution (0, 0, 0, 0 . 0) if k == m: Given an array arr[] of size N, representing the number of balls of each of N distinct colors, the task is to find the probability of distributing all the balls into two boxes, such that both the boxes contain an equal number of distinct colored balls. Every box has at least no balls and at most N balls. Of course the total number of balls in M boxes must equal to N. For each allocation, I calculated a value based on the allocation: V=f(n_1,n_2,.n_m) . Enumerate the ways of distributing the balls into boxes. Some boxes may be empty. We can represent each distribution in the form of n stars and k − 1 vertical lines. The stars represent balls, and the vertical lines divide the balls into boxes. For example, here are the possible distributions for n = 3, k = 3:
Each box has a set of 10 distinct colored balls. The colors are numbered from 1 to 10. Each box has a cost associated with it. She is required to spend the minimum amount of money to buy the boxes such that she has maximum distinct colored balls in the end. Input format.Geek wants to distribute M balls among N children. His nemesis Keeg wants to disrupt his plan and removes P balls from Geek's bag. The minimum number of balls required to make each child happy are given in an array arr[]. Find the number of ways
Now, you can distribute apples from any single pack into multiple boxes if necessary, but what you're trying to find out is the smallest number of boxes you can use to hold all the apples. Imagine you're moving and have a collection of differently sized boxes and many items of .

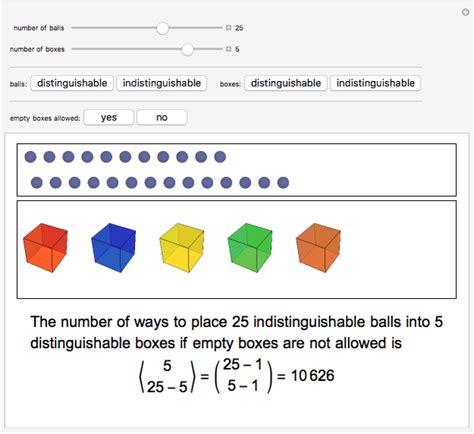
Distributing balls in boxes.

Distributing all balls without repetition
Distributing Balls
Balls in Boxes
Pure metal / Used as an alloy element for aluminum, lead, zinc, and other .
distribution balls into boxes java|Algorithms for allocating N balls into M boxes