describing distribution of box plots skew In this journey through “Box Plot Skewness: Decoding Asymmetry,” we’ve unlocked the secrets of box plots and their role in revealing data distribution. We’ve seen how skewness, whether right, left, or . David Jones Elizabeth Street and Market St Sydney stores will open at 5am on Boxing Day, with the official bell ringing at Elizabeth St. Westfield Bondi Junction. Boxing Day 7am — 9pm
0 · vertical box plot skewness
1 · skewed right box plot
2 · skewed left whisker plot
3 · right skewed box plot vertical
4 · positively skewed box plots
5 · positive skew vs negative boxplot
6 · left skewed plot
7 · box plot negatively skewed
As more and more electric lines are buried in neighborhoods and throughout your local electric co-op’s territory, more big green metal boxes — about the size of a mini fridge — are likely to be seen. These are pad-mounted transformers.
Box plots visually show the distribution of numerical data and skewness by displaying the data quartiles (or percentiles) and averages. Box plots show the five-number summary of a set of data: including the minimum . In this journey through “Box Plot Skewness: Decoding Asymmetry,” we’ve unlocked the secrets of box plots and their role in revealing data distribution. We’ve seen how skewness, whether right, left, or .To determine whether a distribution is skewed in a box plot, look at where the median line falls within the box and whiskers. You have a symmetrical distribution when the box centers approximately on the median line, and the upper and .Create a box plot for the data from each variable and decide, based on that box plot, whether the distribution of values is normal, skewed to the left or skewed to the right, and estimate the value of the mean in relation to the median.
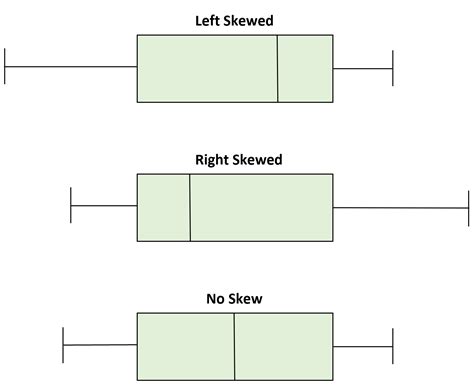
We can determine whether or not a distribution is skewed based on the location of the median value in the box plot. When the median is closer to the bottom of the box and the whisker is shorter on the lower end of the box, .The box plot will look as if the box was shifted to the left so that the right tail will be longer, and the median will be closer to the left line of the box in the box plot. If the distribution is skewed to the left, most values are 'large', but there are a . Two of such metrics are skewness and kurtosis. You can use them to assess the resemblance between your distributions and a perfect, normal distribution. By finishing this article, you will learn in detail: Let’s get started! . Box plots provide basic information about a distribution. For example, a distribution with a positive skew would have a longer whisker in the positive direction than in the negative direction. A larger mean than median .
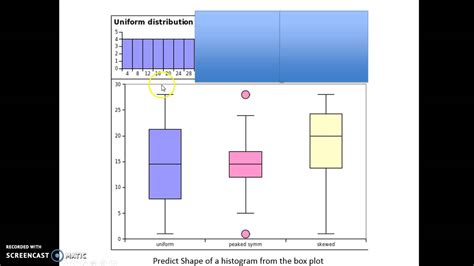
In this post, learn about left and right skewed distributions, how to tell the differences in histograms and boxplots, the implications of these distributions, why they occur, and how to analyze them. Let’s start by contrasting characteristics . We can determine whether or not a distribution is skewed based on the location of the median value in the box plot. When the median is closer to the bottom of the box and the whisker is shorter on the lower end of the box, the distribution is right . Box plots visually show the distribution of numerical data and skewness by displaying the data quartiles (or percentiles) and averages. Box plots show the five-number summary of a set of data: including the minimum score, first (lower) quartile, median, third (upper) quartile, and maximum score. In this journey through “Box Plot Skewness: Decoding Asymmetry,” we’ve unlocked the secrets of box plots and their role in revealing data distribution. We’ve seen how skewness, whether right, left, or symmetrical, provides crucial insights into data characteristics.
To determine whether a distribution is skewed in a box plot, look at where the median line falls within the box and whiskers. You have a symmetrical distribution when the box centers approximately on the median line, and the upper and lower whiskers are about equal length.Create a box plot for the data from each variable and decide, based on that box plot, whether the distribution of values is normal, skewed to the left or skewed to the right, and estimate the value of the mean in relation to the median.
We can determine whether or not a distribution is skewed based on the location of the median value in the box plot. When the median is closer to the bottom of the box and the whisker is shorter on the lower end of the box, the distribution is right .The box plot will look as if the box was shifted to the left so that the right tail will be longer, and the median will be closer to the left line of the box in the box plot. If the distribution is skewed to the left, most values are 'large', but there are a few exceptionally small ones. Two of such metrics are skewness and kurtosis. You can use them to assess the resemblance between your distributions and a perfect, normal distribution. By finishing this article, you will learn in detail: Let’s get started! What is Skewness? Box plots provide basic information about a distribution. For example, a distribution with a positive skew would have a longer whisker in the positive direction than in the negative direction. A larger mean than median would also indicate a positive skew.
In this post, learn about left and right skewed distributions, how to tell the differences in histograms and boxplots, the implications of these distributions, why they occur, and how to analyze them. Let’s start by contrasting characteristics of the symmetrical normal distribution with skewed distributions. We can determine whether or not a distribution is skewed based on the location of the median value in the box plot. When the median is closer to the bottom of the box and the whisker is shorter on the lower end of the box, the distribution is right . Box plots visually show the distribution of numerical data and skewness by displaying the data quartiles (or percentiles) and averages. Box plots show the five-number summary of a set of data: including the minimum score, first (lower) quartile, median, third (upper) quartile, and maximum score.
In this journey through “Box Plot Skewness: Decoding Asymmetry,” we’ve unlocked the secrets of box plots and their role in revealing data distribution. We’ve seen how skewness, whether right, left, or symmetrical, provides crucial insights into data characteristics.To determine whether a distribution is skewed in a box plot, look at where the median line falls within the box and whiskers. You have a symmetrical distribution when the box centers approximately on the median line, and the upper and lower whiskers are about equal length.Create a box plot for the data from each variable and decide, based on that box plot, whether the distribution of values is normal, skewed to the left or skewed to the right, and estimate the value of the mean in relation to the median.
We can determine whether or not a distribution is skewed based on the location of the median value in the box plot. When the median is closer to the bottom of the box and the whisker is shorter on the lower end of the box, the distribution is right .The box plot will look as if the box was shifted to the left so that the right tail will be longer, and the median will be closer to the left line of the box in the box plot. If the distribution is skewed to the left, most values are 'large', but there are a few exceptionally small ones. Two of such metrics are skewness and kurtosis. You can use them to assess the resemblance between your distributions and a perfect, normal distribution. By finishing this article, you will learn in detail: Let’s get started! What is Skewness? Box plots provide basic information about a distribution. For example, a distribution with a positive skew would have a longer whisker in the positive direction than in the negative direction. A larger mean than median would also indicate a positive skew.
cnc processing manufacturer
vertical box plot skewness
skewed right box plot
skewed left whisker plot
Free and open company data on United Kingdom company WESTMINSTER METAL FABRICATORS LTD (company number 14535347), Unit 14, 1-3 Uxbridge Road, Hayes, Middlesex, UB4 0JN
describing distribution of box plots skew|right skewed box plot vertical